1.5 Q-3

Question Statement

Draw the graph of the functions defined below and find whether they are continuous.

i.

$$y = \begin{cases} x - 1 & \text{if } x < 3 2x + 1 & \text{if } x \geq 3 \end{cases}$$

Background and Explanation

This problem involves piecewise functions where the rule for $y$ changes depending on the value of $x$. To determine continuity, we need to check whether there are any discontinuities at the boundary where the function switches from one rule to the other.

Solution

For the first part of the problem, let’s first compute the values for $x$ when $x < 3$ using $y = x - 1$.

$x$	-1	0	1	2
$y = x - 1$	-2	-1	0	1

Points: $(-1,-2), (0,-1), (1,0), (2,1)$

For $x \geq 3$, the function follows $y = 2x + 1$.

$x$	3	4	5	6
$y = 2x + 1$	7	9	11	13

Points: $(3,7), (4,9), (5,11), (6,13)$

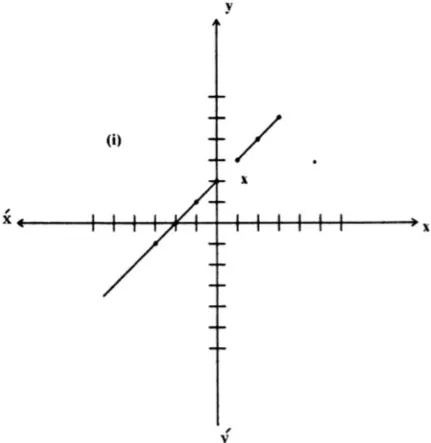
The graph shows two different lines representing the function. Hence, the function is discontinuous at $x = 3$.

Graph:

---

ii. $y = \frac{x^2 - 4}{x - 2}$

Solution

Simplifying the given equation:

$$y = \frac{x^2 - 4}{x - 2} \quad \forall x \in \mathbb{R} \text{ but } x \neq 2$$

Factoring $x^2 - 4$ gives us:

$$y = \frac{(x - 2)(x + 2)}{x - 2} = x + 2 \quad \text{for} \quad x \neq 2$$

Domain: The function is undefined at $x = 2$, so we exclude it from the domain. Let’s prepare the table for some values of $x$ in the domain:

$x$	-4	-3	-2	-1	0	1	1.5	2	2.5	3	4
$y$	-2	-1	0	1	2	3	3.5	—	4.5	5	6

Graph:

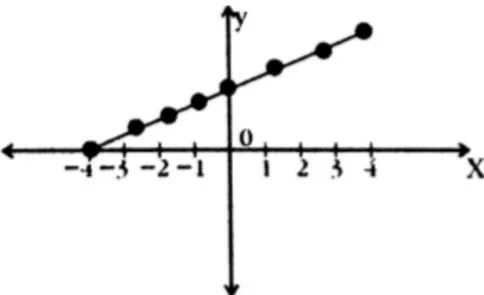
The graph is a straight line with a hole at the point $(2, 5)$, as the function is undefined at $x = 2$. The graph shows a discontinuity at $x = 2$.

---

iii.

$$y = \begin{cases} x + 3 & \text{if } x \neq 3 2 & \text{if } x = 3 \end{cases}$$

Solution

Here, the function follows two different rules. For $x \neq 3$, the function is $y = x + 3$. For $x = 3$, the function is $y = 2$.

We now prepare the table for values of $x$ in the domain:

$x$	-5	-4	-3	-2	-1	0	1	2	3	4	5
$y$	-2	-1	0	1	2	3	4	5	2	7	8

Graph:

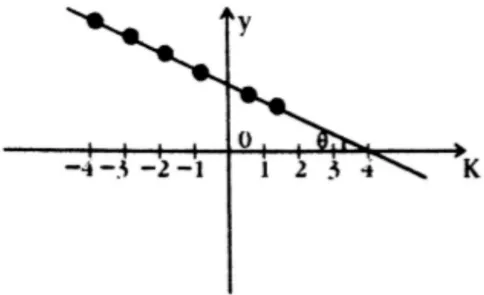
The graph is a straight line with a break at $x = 3$, where the function jumps from $y = 5$ to $y = 2$. This shows a discontinuity at $x = 3$.

---

iv. $y = \frac{x^2 - 16}{x - 4}$

Solution

Simplifying the equation:

$$y = \frac{x^2 - 16}{x - 4} \quad \forall x \in \mathbb{R} \text{ but } x \neq 4$$

Factoring $x^2 - 16$ gives:

$$y = \frac{(x - 4)(x + 4)}{x - 4} = x + 4 \quad \text{for} \quad x \neq 4$$

Domain: The function is undefined at $x = 4$. Let’s prepare the table for some values of $x$ in the domain:

$x$	-4	-3	-2	-1	0	1	2	3.5	4	4.5	5
$y$	0	1	2	3	4	5	6	7.5	—	8.5	9

Graph:

The graph is a straight line with a hole at the point $(4, 8)$, showing a discontinuity at $x = 4$.

---

Key Formulas or Methods Used

1. Piecewise functions: Where different rules are applied to different intervals.
2. Simplification of rational expressions to find the continuous form of the function.
3. Checking continuity by inspecting the graph for breaks or holes at specific points.

Summary of Steps

1. For each function, express the given function in simplified form if possible.
2. Prepare a table with values of $x$ and $y$ for the domain.
3. Plot the points and analyze whether the function has any discontinuities.
4. Verify the graph and determine whether the function is continuous or discontinuous at specific points.

Reference